General Relativity

Lecturer:
Priv.-Doz. Dr. Matteo Maturi (ITA/ZAH, ITP)

Space and time:

• Summer semester, 2022
• From 20.4.22 to 2.8.22
• Monday 09:15-11:00 (INF308/HS 2)
• Wednesday 09:15-11:00 (INF308/HS 2)

Overview:
Gravity is the weakest of all forces in nature and yet it shapes our universe on all scales, from humans bind to Hearth up to defining the dynamics of the entire universe. The lectures will open the path in the understanding on gravity as described by the theory of General Relativity. The students will learn about the properties of flat and curved space-times, the behaviour of massive and massless particles in presence of gravity, black holes a different kinds, gravitational waves, the dynamic of the universe, etc...

Prerequisites:
PTP2, PTP3 is helpful but not mandatory.

Format:
The lectures will be held in person and will be recorded. Lecture notes about what will be present at the blackboard and additional material will be provided. The notes will be complementary to other material/books and present full derivations. I will start slow to build a solid background. The lectures and exercise classes are held in English and will be recorded.

Important:
To get credit points for the lectures it is necessary to enroll, see above.

Material and exercises:
You find everything in this page.

Exam:
WrittenWritten, August 9th, 2022, from 9am to 1pm

Admission to the exam:
Attend at least 50% of the tutorials. If attendance < 50%, it is required to hand in 3 full exercise sheets that will be graded. AND gain 3 points by: presenting half exercise (1 point) actively participating in the discussion during the tutorials (max 1 point per tutorial).

Lehre, Studium und Forschung:
Lecture Token MKTP3.1 (8CP): LSF

 

 

First Ever ray tracing simulation of a black hole (1979 © Jean-Pierre Luminet/CNRS Phototheque)

 

First ever 'picture' of a shadow of a black hole (EHT collaboration)

 

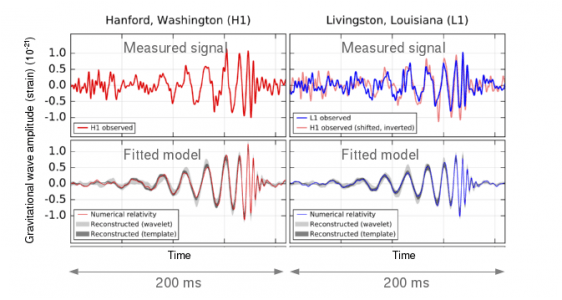
First ever direct detectin of a gravitational wave (Ligo and Virgo collaboration)

Index of the lectures

In blue the parts already covered during the lectures

PART 1: Intro

Newtonian gravity:
    1. Newtonian gravity: idea and problems
      
The equivalence principle:
    1. The equivalence principle,  gravity ↔ non inertial frames
    2. Predictions: gravitational redshift and lensing

More then Newtonian gravity
    2. The most general classical non-relativistic gravitational field
    3. The link between Φ α r-1 and the Euclidean space

PART 2: flat space-time


Special relativity: Minkowski space-time
    1. Special relativity, the need, the idea and the the Lorentz transforms
    2. The Lorentz geometry and the Lorentz group
    3. Groups, Lie-groups, Lie algebra applied to the Lorentz transformation
    4. Relativistic mechanics

Attempting a relativistic linear theory of gravity
    1. Dynamic of the field
    2. Dynamic of a particle in the field: perihelion shift problem

Approaching general relativity: gravity ↔ non inertial frames
    1. Recalling the equivalence principle
    2. Non-inertial frames and the equivalence principle: example, a rotating frame
    3. Connection between gravity and the metric of space-time

PART 3: curved space-time

Curved space-time
    1. Getting formal: scalars, vectors, one-forms and tensors
    2. Manifolds, geometry, Riemanian geometry
    3. The tangent space
    4. Connection and covariant derivatives
    5. Link between the connection and the metric tensor
    6. Parallel transport and the geodesic equations
    7. Conserved quantities and killing vectors
    8. Curvature: the Riemann tensor
    9. Geodesic deviation equation
    10. Strong-equivalence principle; electrodynamics in curved space-time

Field equations
    1. Einstein field equations, Einstein's approach
    2. Einstein field equations, Hilbert's approach
    3. Energy-momentum tensor of matter
    4. Is there one single theory of gravity?
    5. Linearized field equations

PART4: applications

Gravitational waves
    1. Gravitational waves
    2. Generation of gravitational waves

Spherically symmetric systems
    1. Schwartzshild metric
    2. Schwartzshild black-holes
    2. Schwartzshild black-holes
    4. Kruskal coordinates
    5. Reissner-Nordström (electrically charged black-holes)
    6. Kerr metric (rotating spherical objects)

Cosmology, isotropic and homogeneous universe
    1. Friedmann(-Lamaitre)-Robertson-Walker metric (FLRW)
    2. distances
    3. the expansion of the universe
    4. cosmological redshift / energy “non conservation”
    5. The cosmological constant and dark energy

A pinch of numerical general relativity
    1. Numerical simulations of black holes accretion
    2. Cosmological numerical simulations

 

NEW: Lectures/tutorials evaluation
   please fill in this form: https://uni-heidelberg.evasys.de/evasys/online.php?p=C2U9Y